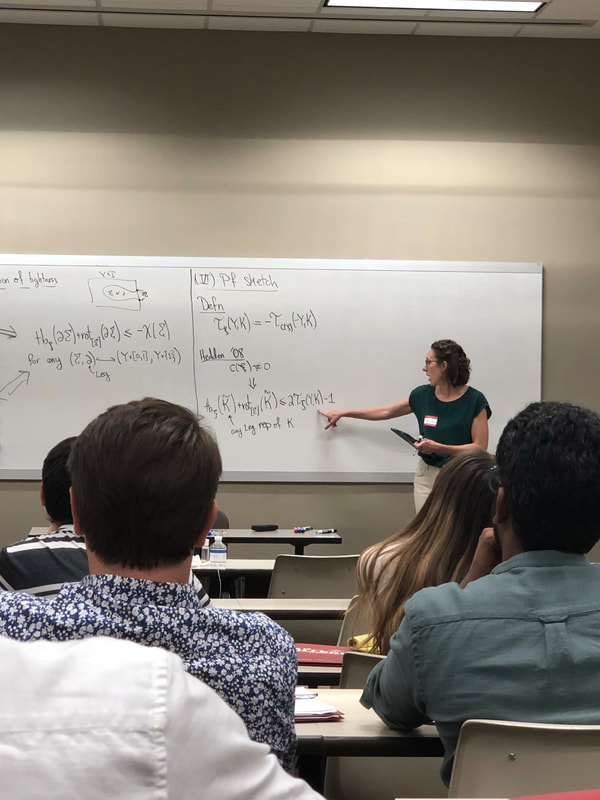SEC Geometry and Topology Workshop
June 2-4, University of Alabama
Goal
Welcome to the first SEC Topology Workshop. This will be an annual workshop series that aims to bring together active researchers, ranging from leading experts to recent PhDs and graduate students to discuss some common research in modern geometry and topology. The main participants and speakers will be researchers at some of the SEC institutions. The workshop will include three mini lecture series, problem sessions, research talks and professional development activities.
Mini Lectures
- Spinal Open Books by Jeremy Van Horn-Morris (Arkansas) and Sam Lisi (Mississippi)
- Lagrangian Cobordisms and Fillings by Angela Wu (Louisiana State) and Shea Vela-Vick (Louisiana State)
- Tightness and 4D Adjunction Inequalities by Katherine Raoux (Arkansas)
Other Research Talks
- John Etnyre (Ga Tech)
- Justin Murray (Louisiana State)
- Nur Saglam (Georgia Tech)
- Agniva Roy (Georgia Tech and Louisiana State)
Registration and support
Some funding is available for workshop participants. To register and request funding please email Bulent Tosun at [email protected]. The deadline to request funding is May 1.
The workshop is sponsored by the National Science Foundation Grants DMS 2144363, DMS 2105525 and the Simons Foundation.
The workshop is sponsored by the National Science Foundation Grants DMS 2144363, DMS 2105525 and the Simons Foundation.
Local Information and Venue
Accommodations:
Restaurants:
Venue: All the talks will be in Gordon Palmer (GP 208) where the mathematics department is located. See the campus map
- Capstone Inn--a few minutes walking distance to the math department.
- Hampton Inn -- 15-20 minutes walking distance to the math department.
Restaurants:
- Many restaurant options in the strip area which is in 10-15 minutes walking distance from the math department.
- Archibald's and Dreamland are well-known/authentic BBQ options in Tuscaloosa
- River, Chuck's Fish, Five, Central Mesa, Avenue Pub, Evangeline's, Antojitos Izcalli are some good restaurants in Tuscaloosa.
Venue: All the talks will be in Gordon Palmer (GP 208) where the mathematics department is located. See the campus map
Schedule
|
Friday
8:30-9:30: Check-in 9:30-10:30 Lecture Series 1a: Jeremy Van Horn Morris/ Sam Lisi 10:30-11:00 Break--Coffee/Snacks from Heritage House 11:00-12:00 Lecture Series 1b: Sam Lisi/Jeremy Van Horn-Morris 12:00-1:30 Lunch--Dreamland BBQ 1:30-2:15 Research Talk: Agniva Roy 2:30-3:30 Lecture Series 2a: Katherine Raoux 3:30-4:00 Break 4:00-5:00 Lecture Series 2b: Katherine Raoux 5:30-Happy hour at the Venue |
Saturday
9:30-10:30 Lecture Series 3a: Shea Vela-Vick 10:30-11:00 Break--Coffee/Snacks from Heritage House 11:00-12:00 Lecture Series 3b: Angela Wu 12:00-1:30 Lunch--Sandwiches from Newk's 1:30-2:15 Research Talk: John Etnyre 2:30-3:00 Break 3:00-3:45 Research Talk: Nur Saglam 4:00-5:00 Professional Development Activity Job Process for Mathematicians by Etnyre (Slides) 6:00-8:00 Conference Meal at the River |
Sunday
9:30-10:30 Discussion/Breakout Session 10:30-11:00 Break--bagels, donuts and coffee 11:00-11:30 Short Talk: Justin Murray 11:30-12:15 Planning SEC Topology 2024 |
Participants
- Bruce Trace (Alabama)
- Bulent Tosun (Alabama)
- Lawrence Roberts (Alabama)
- Jon Carson (Alabama)
- Thomas Polstra (Alabama)
- Kyungyong Lee (Alabama)
- Martyn Dixon (Alabama)
- Martin Evans (Alabama)
- Uly Alvarez (Alabama)
- Tucker Ervin (Alabama)
- Courtenay Morse (Alabama)
- Nicole Bruner (Alabama)
- Evan Lee (Alabama)
- Puji Das (Alabama)
- Shea Vela-Vick (Louisiana State)
- Angela Wu (Louisiana State)
- Scott Baldridge (Louisiana State)
- Colton Sandvik (Louisiana State)
- Nilangshu Bhattacharyya (Louisiana State)
- Justin Murray (Louisiana State)
- Megan Farrell (Louisiana State)
- Kiran Bist (Louisiana State)
- Amit Kumar (Louisiana State)
- Adithyan Pandikkadan (Louisiana State)
- Jeremy Van Horn-Morris (Arkansas)
- Katherine Raoux (Arkansas)
- Kevin Tuttle (Arkansas)
- Aidan McCue (Arkansas)
- Sam Lisi (Mississippi)
- Joe Lopez (Mississippi)
- John Etnyre (Georgia Tech)
- Colby Shaw (Georgia Tech)
- Agniva Roy (Georgia Tech and Louisiana State)
- Nur Saglam (Georgia Tech)
- Audrick Pyronneau (UWA and UT Austin)
- Nico Fontova (UGA and NC Sate)
- Veronica King (UT Austin)
Titles and Abstracts
Sam Lisi/Jeremy Van Horn-Morris
Title: Spinal Open Books
Abstract: This sequence of talks will start with a discussion of open book decompositions for contact 3-manifolds and their connection to Lefschetz fibrations over a disk. We will then introduce a discussion of Lefschetz fibrations over a more general surface and see this as a motivation for the definition of a spinal open book decomposition. Finally, we will see why this definition is useful, first with a brief introduction to using J-holomorphic curves to study symplectic fillings of a contact manifold, followed by some applications of spinal open book decompositions to understanding contact 3-manifolds and their fillings.
Katherine Raoux
Title:Tightness and 4D Adjunction Inequalities
Abstract: Surfaces with Legendrian boundary in tight contact 3-manifolds satisfy the Eliashberg-Bennequin inequality. This inequality constrains the Euler characteristic of the surface in terms of invariants of the boundary knot. In the tight 3-sphere, Rudolph showed the same Bennequin bound constrains the genus of surfaces embedded 4-ball with Legendrian boundary. Key to his methods is Kronheimer and Mrowka’s adjunction inequality.
In the first lecture we discuss a relative version of the adjunction inequality and its relationship to Heegaard Floer theoretic tau-invariants. The second lecture applies relative adjunction, extending Rudolph’s result to a larger class of contact 3-manifolds. Finally, we present several conjectures that, if proved, would fully establish a 4-dimensional characterization of tightness.
This is based on joint work with Matthew Hedden.
Angela Wu/Shea Vela-Vick
Title: Lagrangian Cobordisms and Fillings
Abstract:
Legendrian knots are smooth knots which are compatible with an ambient contact structure. They are an essential object of study in contact and symplectic geometry, and many easily posed questions about these knots remain unanswered. Two smooth knots are said to be cobordant if they jointly form the boundary of a surface in four-dimensional Euclidean space. In the symplectic setting, we say they are Lagrangian cobordant if the knots are Legendrian and the surface is Lagrangian. Interestingly, Lagrangian cobordism is, unlike smooth cobordism, not a symmetric relation. If the Legendrian knot on the negative end is the empty one, we call this a Lagrangian filling of the Legendrian knot on the positive end. A lot of recent work has been done towards understanding both Lagrangian cobordisms and fillings. In this lecture series, we’ll properly define Lagrangian cobordisms and fillings, discuss their known properties, and talk about various strategies that can be used to construct and obstruct them.
Justin Murray
Title: The Homotopy Cardinality of the Representation Category
Abstract: Given a Legendrian knot in $(\mathbb{R}^3, \ker(dz-ydx))$ one can assign a combinatorial invariants called ruling polynomials. These invariants have been shown to recover not only a (normalized) count of augmentations but are also closely related to a categorical count of augmentations in the form of the homotopy cardinality of the augmentation category. In this talk, we will introduce the homotopy cardinality of the $n$-dimensional representation category and establish its relation to the $n$-colored ruling polynomial. Along the way, we establish that two $n$-dimensional representations are equivalent in the representation category iff they are ``conjugate DGA homotopic''. We also provide some applications to Lagrangian concordance.
John Etnyre
Title: Non-loose knots: existence, construction, and classification.
Abstract: I will discuss necessary and sufficient conditions for a knot type to admit Legendrian, or transverse, representatives in an overtwisted contact structure that have tight complements (these are called non-loose knots). In the process we will need to give the classification of all non-loose rational unknots, rational unknots are corse of Heegaard tori in lens spaces. If time permits, we will also discuss how non-looseness interacts with cabling. This is joint work with Chatterjee, Min, and Mukherjee.
Agniva Roy
Title: Small caps for Lens spaces and handlebody descriptions of CP^2
Abstract: Using mutations of almost toric fibrations, Vianna and Evans-Smith constructed embeddings of lens spaces into CP^2. The associated combinatorics has deep connections to mirror symmetry and cluster algebras. Using work of Gay from early 2000s, and inspiration from recent work of Etnyre-Min-Mukherjee on non-loose knots in overtwisted contact structures, we reinterpret the almost toric pictures as symplectic handlebody descriptions of CP^2. Further, we discover that the related combinatorics have an alternate, and possibly related, description in terms of fractions on the Farey graph. This is joint work with Etnyre, Min, and Piccirillo.
Nur Saglam
Title: Fillability of Contact Structures on the 3-manifolds obtained by surgeries on the trefoil knot
Abstract: Let M be the 3-manifold obtained by r-surgery on the right handed trefoil knot. Classification of contact structures on such manifolds have been mostly understood for r\geq 1 and r=0. Etnyre-Min-Tosun has an upcoming work on the classification of the tight contact structures for all r. The fillability of contact structures on M is mostly understood if r is not between 0 and 1/2. In this talk, we will discuss the fillability of the contact structures M for 0<r<1/2. How many contact structures does M admit? How many of them are strongly fillable but not Stein fillable? This is a joint work with John Etnyre.
Title: Spinal Open Books
Abstract: This sequence of talks will start with a discussion of open book decompositions for contact 3-manifolds and their connection to Lefschetz fibrations over a disk. We will then introduce a discussion of Lefschetz fibrations over a more general surface and see this as a motivation for the definition of a spinal open book decomposition. Finally, we will see why this definition is useful, first with a brief introduction to using J-holomorphic curves to study symplectic fillings of a contact manifold, followed by some applications of spinal open book decompositions to understanding contact 3-manifolds and their fillings.
Katherine Raoux
Title:Tightness and 4D Adjunction Inequalities
Abstract: Surfaces with Legendrian boundary in tight contact 3-manifolds satisfy the Eliashberg-Bennequin inequality. This inequality constrains the Euler characteristic of the surface in terms of invariants of the boundary knot. In the tight 3-sphere, Rudolph showed the same Bennequin bound constrains the genus of surfaces embedded 4-ball with Legendrian boundary. Key to his methods is Kronheimer and Mrowka’s adjunction inequality.
In the first lecture we discuss a relative version of the adjunction inequality and its relationship to Heegaard Floer theoretic tau-invariants. The second lecture applies relative adjunction, extending Rudolph’s result to a larger class of contact 3-manifolds. Finally, we present several conjectures that, if proved, would fully establish a 4-dimensional characterization of tightness.
This is based on joint work with Matthew Hedden.
Angela Wu/Shea Vela-Vick
Title: Lagrangian Cobordisms and Fillings
Abstract:
Legendrian knots are smooth knots which are compatible with an ambient contact structure. They are an essential object of study in contact and symplectic geometry, and many easily posed questions about these knots remain unanswered. Two smooth knots are said to be cobordant if they jointly form the boundary of a surface in four-dimensional Euclidean space. In the symplectic setting, we say they are Lagrangian cobordant if the knots are Legendrian and the surface is Lagrangian. Interestingly, Lagrangian cobordism is, unlike smooth cobordism, not a symmetric relation. If the Legendrian knot on the negative end is the empty one, we call this a Lagrangian filling of the Legendrian knot on the positive end. A lot of recent work has been done towards understanding both Lagrangian cobordisms and fillings. In this lecture series, we’ll properly define Lagrangian cobordisms and fillings, discuss their known properties, and talk about various strategies that can be used to construct and obstruct them.
Justin Murray
Title: The Homotopy Cardinality of the Representation Category
Abstract: Given a Legendrian knot in $(\mathbb{R}^3, \ker(dz-ydx))$ one can assign a combinatorial invariants called ruling polynomials. These invariants have been shown to recover not only a (normalized) count of augmentations but are also closely related to a categorical count of augmentations in the form of the homotopy cardinality of the augmentation category. In this talk, we will introduce the homotopy cardinality of the $n$-dimensional representation category and establish its relation to the $n$-colored ruling polynomial. Along the way, we establish that two $n$-dimensional representations are equivalent in the representation category iff they are ``conjugate DGA homotopic''. We also provide some applications to Lagrangian concordance.
John Etnyre
Title: Non-loose knots: existence, construction, and classification.
Abstract: I will discuss necessary and sufficient conditions for a knot type to admit Legendrian, or transverse, representatives in an overtwisted contact structure that have tight complements (these are called non-loose knots). In the process we will need to give the classification of all non-loose rational unknots, rational unknots are corse of Heegaard tori in lens spaces. If time permits, we will also discuss how non-looseness interacts with cabling. This is joint work with Chatterjee, Min, and Mukherjee.
Agniva Roy
Title: Small caps for Lens spaces and handlebody descriptions of CP^2
Abstract: Using mutations of almost toric fibrations, Vianna and Evans-Smith constructed embeddings of lens spaces into CP^2. The associated combinatorics has deep connections to mirror symmetry and cluster algebras. Using work of Gay from early 2000s, and inspiration from recent work of Etnyre-Min-Mukherjee on non-loose knots in overtwisted contact structures, we reinterpret the almost toric pictures as symplectic handlebody descriptions of CP^2. Further, we discover that the related combinatorics have an alternate, and possibly related, description in terms of fractions on the Farey graph. This is joint work with Etnyre, Min, and Piccirillo.
Nur Saglam
Title: Fillability of Contact Structures on the 3-manifolds obtained by surgeries on the trefoil knot
Abstract: Let M be the 3-manifold obtained by r-surgery on the right handed trefoil knot. Classification of contact structures on such manifolds have been mostly understood for r\geq 1 and r=0. Etnyre-Min-Tosun has an upcoming work on the classification of the tight contact structures for all r. The fillability of contact structures on M is mostly understood if r is not between 0 and 1/2. In this talk, we will discuss the fillability of the contact structures M for 0<r<1/2. How many contact structures does M admit? How many of them are strongly fillable but not Stein fillable? This is a joint work with John Etnyre.